This worksheet is designed to help students practice and understand basic fractions-related concepts. Let’s break down its purpose and content:
Purpose:
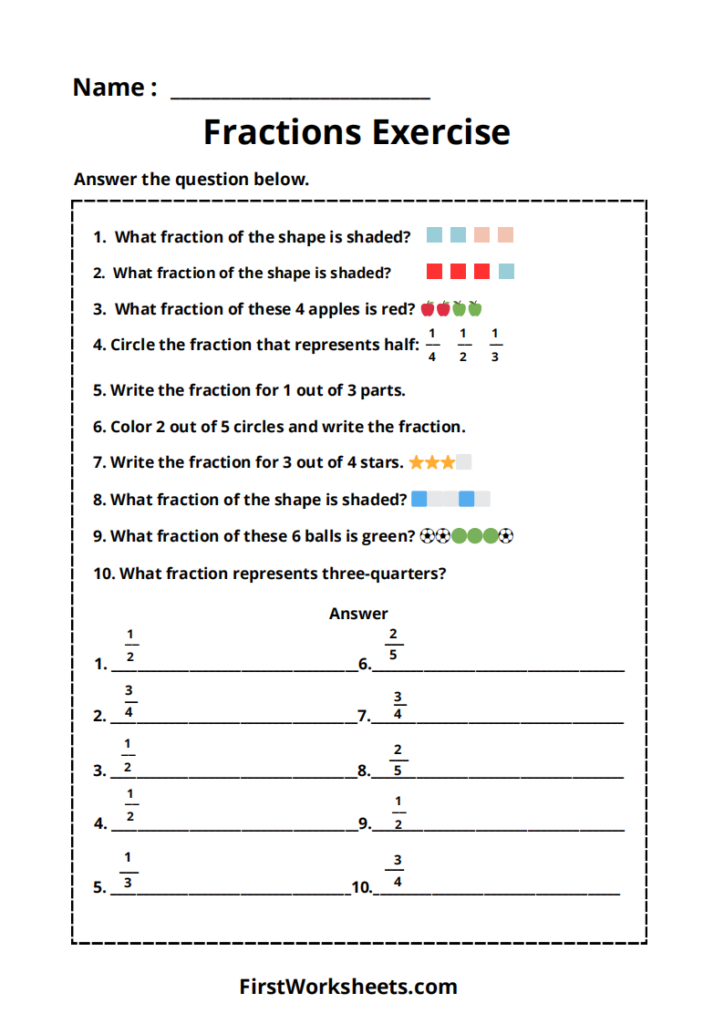
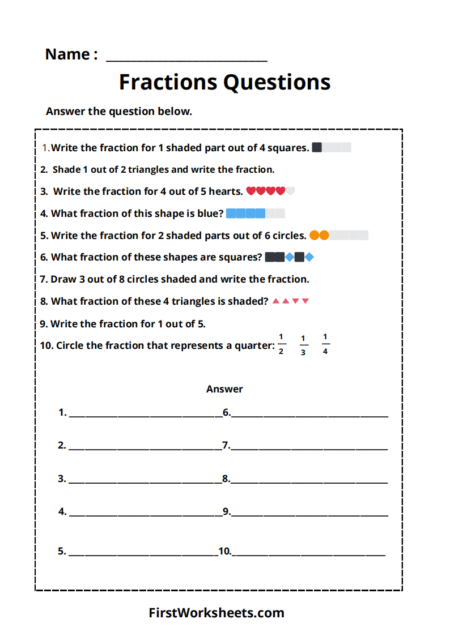
- Introduction to Fractions: The worksheet introduces the concept of fractions as parts of a whole.
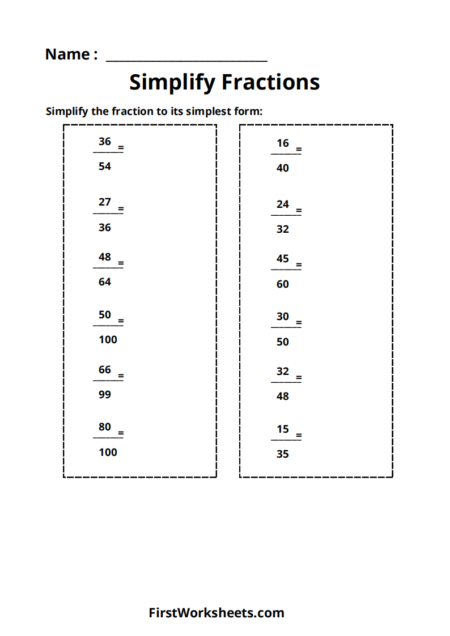
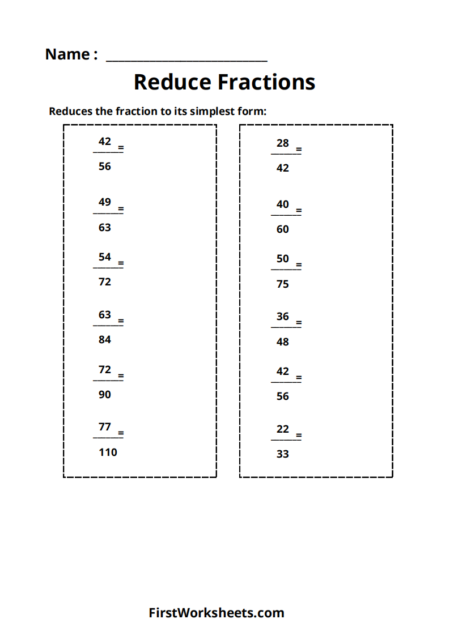
- Fraction Representation: It helps students understand how fractions are represented numerically (e.g., 1/2, 3/4) and visually through shaded shapes or parts of a group of objects.
- Identifying Fractions: Students are asked to identify the fraction represented by shaded areas in various shapes.
- Relating Fractions to Real-Life Objects: The worksheet uses examples like apples and balls to help students connect the concept of fractions to everyday objects.
- Understanding Basic Fraction Terminology: It introduces terms like “half,” “quarter,” and “three-quarters.”
Content:
- Questions with Visuals: The worksheet includes questions that ask students to determine the fraction of a shape that is shaded. This helps them visualize fractions and understand the relationship between the shaded part and the whole.
- Questions with Groups of Objects: Students are asked to identify the fraction of a group of objects (e.g., red apples out of total apples) that have a certain characteristic. This helps them apply the concept of fractions to sets of items.
- Fraction Representation: Students are asked to write the numerical representation of a fraction based on a description (e.g., “1 out of 3 parts”).
- Coloring Activity: The worksheet includes a coloring activity where students color a specific number of objects and then write the corresponding fraction. This reinforces the connection between the visual representation and the numerical representation of a fraction.
- Multiple-Choice Question: There is a question asking students to circle the fraction that represents “half.” This helps assess their understanding of this common fraction.
This worksheet provides a foundational introduction to fractions, helping students understand how fractions represent parts of a whole, how they are written numerically, and how they can be applied to real-life situations.
Answer Key