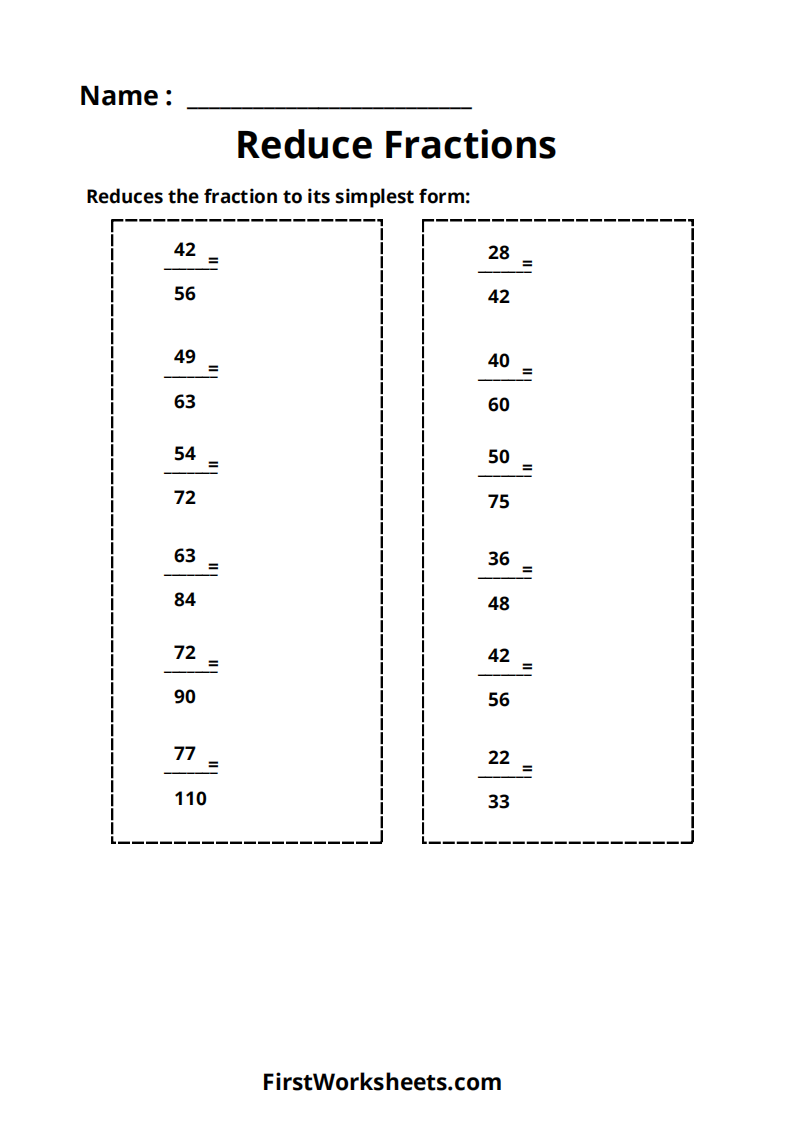
This worksheet focuses on simplifying or reducing fractions.
Purpose:
- Understanding Equivalent Fractions: The core goal is to teach students that fractions can be represented in multiple forms while maintaining the same value. For instance, the fraction 1/2 is equivalent to 2/4, 3/6, and so on.
- Finding the Simplest Form: The worksheet emphasizes reducing fractions to their “simplest form.” This means expressing the fraction with the smallest possible numerator and denominator. This is achieved by dividing both the top (numerator) and bottom (denominator) numbers by their Greatest Common Divisor (GCD).
- Building Number Sense: Working with fractions in this way helps students develop a stronger sense of number relationships and improves their ability to perform operations with fractions later on.
Worksheet Structure:
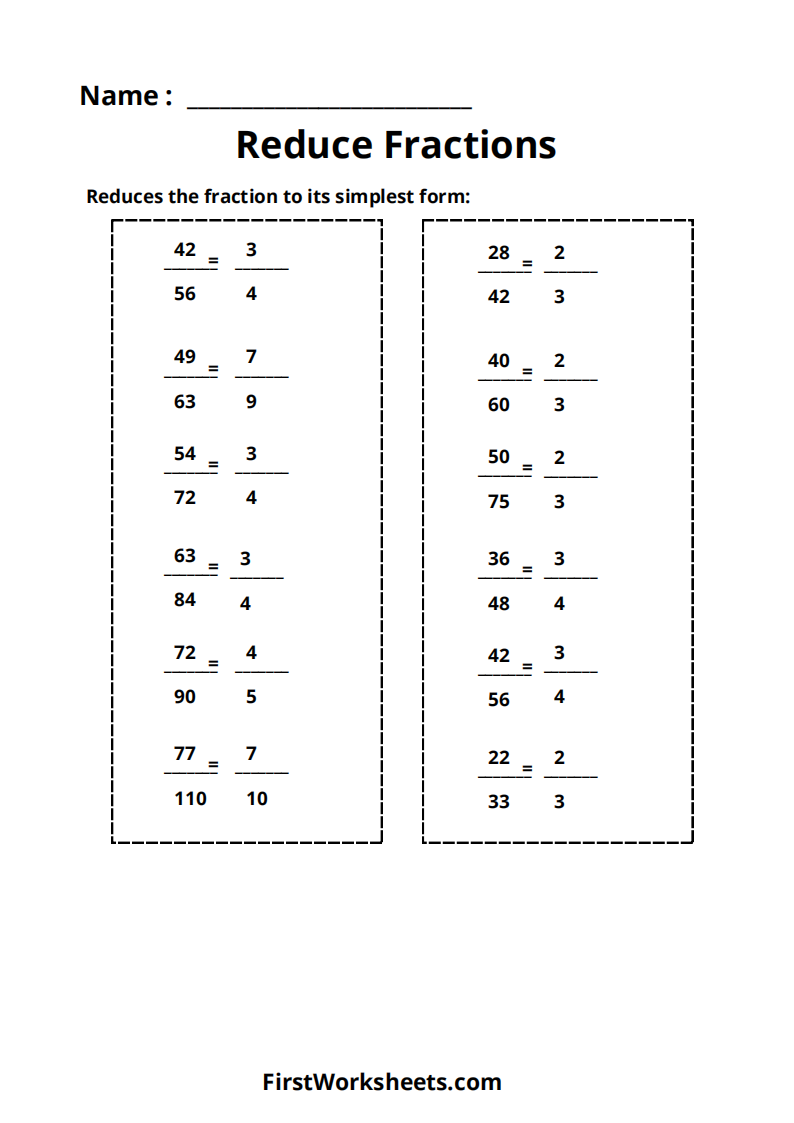
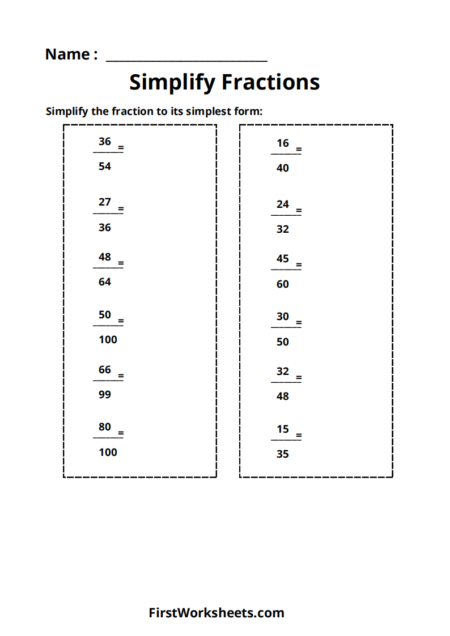
- Problems: The worksheet provides a list of fractions.
- Solutions: The second page shows the same fractions with their reduced or simplified forms.
- Process (Implied): Students are expected to find the GCD of the numerator and denominator of each fraction and then divide both by this GCD to arrive at the simplest form.
Example:
- Fraction: 42/56
- GCD of 42 and 56 is 14.
- Dividing both numerator and denominator by 14: 42/14 = 3 and 56/14 = 4.
- Simplified Form: 3/4
This worksheet is a foundational tool for teaching students how to work with fractions effectively.
Answer Key