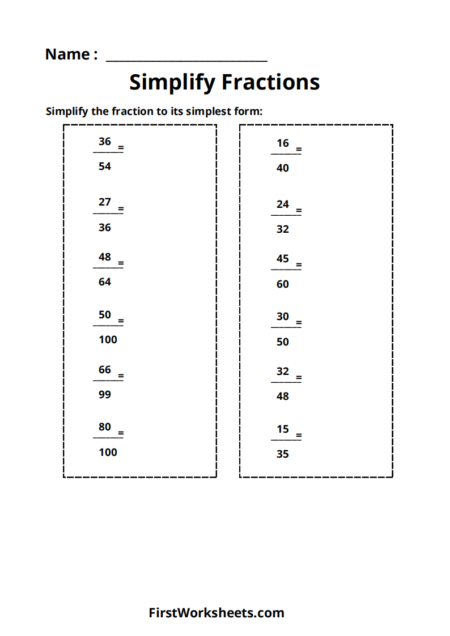
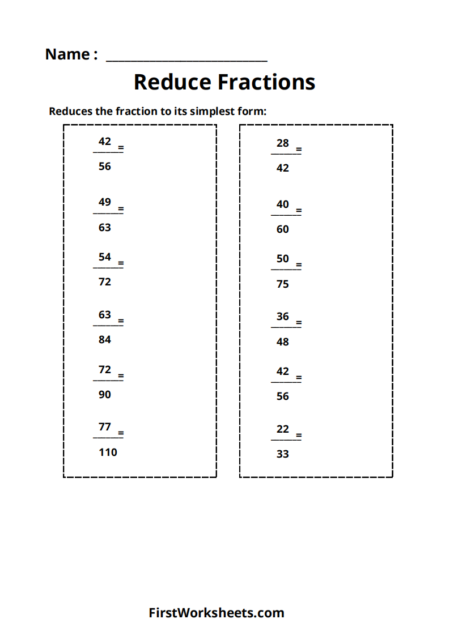
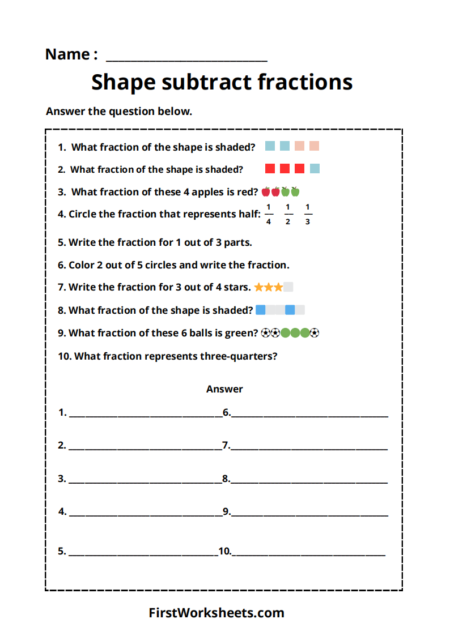
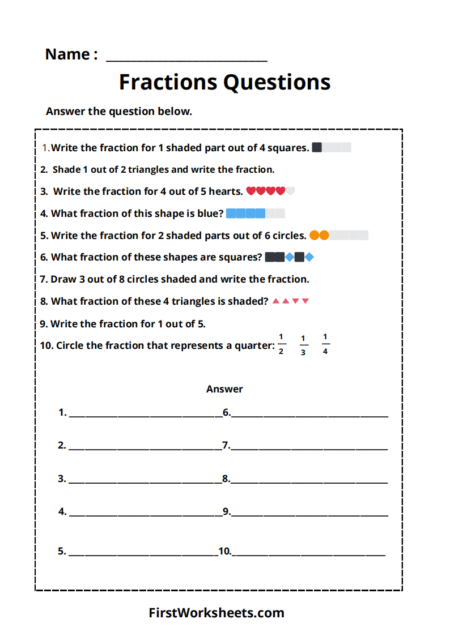
This worksheet focuses on simplifying or reducing fractions to their simplest form. This means expressing the fraction in its lowest terms, where the numerator (top number) and denominator (bottom number) have no common factors greater than 1.
Purpose:
- Understanding Fraction Equivalence: Simplifying fractions helps students recognize that fractions with different numbers can represent the same value (e.g., 1/2 is the same as 2/4).
- Mastering Basic Math Skills: This skill is essential for further math topics like adding, subtracting, multiplying, and dividing fractions.
- Improving Problem-Solving: Simplifying fractions often makes calculations easier and less prone to errors.
- Real-World Application: Fractions are used in everyday life for cooking, measuring, and various other practical tasks.
Worksheet Structure:
- Problems: The worksheet provides a list of fractions.
- Task: Students are asked to “reduce” or “simplify” each fraction.
- Solutions: The second page shows the original fractions with their simplified forms. For example, the fraction 10/20 is reduced to 1/2.
How to Simplify Fractions:
- Find the Greatest Common Factor (GCD): The GCD is the largest number that divides into both the numerator and denominator without leaving a remainder.
- Divide: Divide both the numerator and denominator by their GCD.
- Result: The resulting fraction is the simplified form.
Example:
- Fraction: 12/16
- GCD: 4 (because 4 is the largest number that divides evenly into both 12 and 16)
- Division: 12 ÷ 4 = 3, 16 ÷ 4 = 4
- Simplified Form: 3/4
This worksheet is a valuable tool for students to practice and improve their fraction simplification skills, which are fundamental for success in mathematics and real-world applications.

Answer Key